I. Fundamentals
16 Intervals
Chelsey Hamm and Bryn Hughes
Key Takeaways
- Two pitches form an interval, which is usually defined as the distance between two notes.
- Melodic intervals are played or sung separately, while harmonic intervals are played or sung together.
- Every interval has a size and a quality. An interval’s size is the distance between two notes on a staff—i.e., it is a measurement of the number of lines and spaces between two notes.
- Size is considered generic. In other words, it doesn’t matter what accidentals you apply to the notes, the size is always the same.
- A quality makes an interval specific when used in combination with a size. Unisons, fourths, fifths, and octaves form perfect intervals, while seconds, thirds, sixths, and sevenths form major and minor intervals.
- Any interval can be augmented or diminished. Augmented intervals are one half step larger than a perfect or major interval. Diminished intervals are one half step smaller than a perfect or minor interval.
- Intervals between a unison and an octave are called simple intervals. Any interval larger than an octave is a compound interval.
- Intervallic inversion occurs when two notes are “flipped.” When you need to identify an interval where the lower note is the tonic of a difficult or imaginary major key, inverting the interval can help.
- Consonant intervals are intervals that are considered more stable, as if they do not need to resolve, while dissonant intervals are considered less stable, as if they do need to resolve.
Two pitches form an interval, which is usually defined as the distance between two notes. But what does an interval measure? Physical distance on the staff? Difference in wavelength between pitches? Something else? Music theorists have had contradictory ideas on the definition of “interval,” and these definitions have varied greatly with milieu. This chapter will focus on intervals as a measure of two things: written distance between two notes on a staff, and an aural “distance” (or space) between two sounding pitches. It will be important to keep in mind at all times that intervals are both written and aural, so that you are thinking of them musically (and not simply as an abstract concept that you are writing and reading).
Size
Intervals can be melodic (played or sung separately) or harmonic (played or sung together). In Example 1, the notes in the first measure sound together (harmonically), while in the second measure, they sound separately (melodically).
https://musescore.com/user/32728834/scores/8418647/s/H5aOaq/embed
Example 1. A harmonic and a melodic interval.
Every interval has a size and a quality. A size is the distance between two notes on a staff—i.e., it is a measurement of the number of lines and spaces between two notes. Sizes are written with Arabic numbers (2, 3, 4, etc.); however, they are spoken with ordinal numbers (second, third, fourth, fifth, sixth, seventh, etc.). Always begin with “one” when counting size. Example 2 shows the eight sizes within a C major scale. As you can see, the sizes are labeled with ordinal numbers, with two exceptions: the interval between two notes on the same line or space is called a “unison,” not a “first,” and notes eight lines and spaces apart are said to be an “octave,” not an “eighth.”
https://musescore.com/user/32728834/scores/8418638/s/e4jxEI/embed
Example 2. Sizes of intervals.
Size is considered generic. In other words, it doesn’t matter what accidentals you apply to the notes—the size is always the same. Example 3 demonstrates this: despite the different accidentals, each of these intervals is a third (or “generic third”) because there are three lines/spaces between the two notes.
https://musescore.com/user/32728834/scores/8418665/s/n71TMI/embed
Example 3. Accidentals do not affect an interval’s generic size.
Perfect, Major, and Minor Qualities
A quality makes an interval specific when used in combination with a size. Quality more precisely measures written distance between notes, and—in combination with an interval’s size—it describes the aural sound of an interval.
There are five possible interval qualities:
- Augmented (designated as A or +)
- Major (ma)
- Perfect (P)
- Minor (mi)
- Diminished (d or o)
The quality comes before the size when saying or writing an interval. For example, an interval could be described as a “perfect fourth” (abbreviated P4), a “minor third” (abbreviated mi3), or an “augmented second” (abbreviated +2 or A2).
For now, we will only discuss three qualities: perfect, major, and minor. Different theorists (in different locations and time periods) have applied these qualities to different sizes of intervals, depending on milieu. Example 4 shows how these qualities are applied today. The left column shows that seconds, thirds, sixths, and sevenths are major and/or minor, while the right column shows that unisons, fourths, fifths, and octaves are perfect intervals.
[table “61” not found /]
Example 4. Interval qualities.
The “Major Scale” Method for Determining Quality
There are several different methods for learning to write and identify qualities of intervals. One method you may have heard of is counting half steps. We do not recommend this method, because it is time consuming and often inaccurate. Instead, we recommend using what you know about major scales to identify interval quality.
To identify an interval (size and quality) using this method, complete the following steps:
- Determine size (by counting lines and spaces between the notes).
- Imagine that the bottom note of the interval is the tonic of a major scale.
- Determine whether or not the top note is in the bottom note’s major scale (imagined in step 2) and assign the corresponding quality.
- If it is: the interval is perfect (if it is a unison, fourth, fifth, or octave) or major (if it is a second, third, sixth, or seventh). If it is not: the interval could be minor (a lowered second, third, sixth, or seventh), or it could be augmented or diminished, which will be covered in the next section.
Example 5 shows two intervals. Try identifying their size and quality:
https://musescore.com/user/32728834/scores/6836733/s/wud8Ld/embed
Example 5. Two intervals.
In Example 5a, the notes are F and C in treble clef. Here is how you would use the “Major Scale” method to identify the interval:
- First, this interval is a generic fifth (F to itself is 1; to G is 2; to A is 3; to B is 4; to C is 5).
- Second, C is within the key of F major (which has one flat, B♭).
- Therefore, the interval is a perfect fifth.
Let’s now use this process for Example 5b. The notes in this example are E♭ and C♭ in treble clef. Let’s go through the same process again:
- First, this interval is a generic sixth (E♭ to itself is 1; to F is 2; to G is 3; to A is 4; to B is 5; to C is 6).
- Second, C♭ is not in the key of E♭ major (which has three flats: B♭, E♭, and A♭).
- Therefore, this is a minor sixth. If it were a major sixth, then the C would have to be C♮ instead of C♭, because C♮ is in the key of E♭ major.
Augmented and Diminished Qualities
To review, there are five possible interval qualities, of which we have covered major, minor, and perfect:
- Augmented (designated as A or +)
- Major (ma)
- Perfect (P)
- Minor (mi)
- Diminished (d or o)
Augmented intervals are one half step larger than a perfect or major interval. The first measure of Example 6a first shows the notes F and C, which form a perfect fifth (because C is in the key of F major). The top note of this interval is then raised by a half step to a C♯, making the interval one half step larger. The interval from F to C♯ is therefore an augmented fifth (abbreviated as either A5 or +5). In the second measure of Example 6a, the first interval is a major sixth between G and E (because E is in the key of G major). The top note is then raised by a half step to E♯, making the interval into an augmented sixth (A6 or +6). The bottom note of an interval can be altered as well. In the first measure of Example 6b, the perfect fifth F–C is turned into an augmented fifth by lowering the F by a half step to F♭, which makes the interval one half step larger than a perfect fifth. In the second measure of Example 6b, the major sixth G–E is turned into an augmented sixth by lowering the G by a half step to G♭.
https://musescore.com/user/32728834/scores/8418677/s/-x_9Yz/embed
Example 6. Augmented intervals created by (a) raising the top note and (b) lowering the bottom note.
Diminished intervals are one half step smaller than a perfect or minor interval. In the first measure of Example 7a, the perfect fifth F–C is made a half step smaller by lowering the top note to C♭, forming a diminished fifth (also called a tritone, usually abbreviated as d5 or o5). In the second measure, G–E form a major sixth, which becomes a minor sixth when the top note is lowered by a half step. The minor sixth then becomes a diminished sixth when the top note is lowered again to E𝄫. Note that contracting an interval by one half step turns perfect and minor intervals into diminished intervals, but it turns major intervals into minor intervals. Again, it is not always the top note that is altered. In Example 7b, the perfect fifth F–C becomes diminished when the bottom note moves up a half step to F♯. In the second measure, the major sixth G–E first becomes a minor sixth when the G moves up a half step to G♯. This minor interval then becomes diminished when the G♯ moves to G𝄪 in the third measure, further contracting the interval by another half step.
https://musescore.com/user/32728834/scores/6836745/embed
Example 7. Diminished intervals created by (a) lowering the top note and (b) raising the bottom note.
Example 8 again demonstrates and summarizes the relative size of intervals. Each bracket in this example is one half step larger or smaller than the brackets to its right and left. In Example 8a, the interval quality is changed by altering the top note with accidentals. As you can see, intervals one half step larger than perfect or major intervals are augmented; intervals one half step smaller than major intervals are minor; and intervals one half step smaller than perfect or minor intervals are diminished. Example 8b outlines the same qualities as 10a, only with the bottom note altered by accidentals instead of the top note.
https://musescore.com/user/32728834/scores/8418728/s/SPtiOU/embed
Example 8. Relative size of intervals with (a) the top note altered and (b) the bottom note altered.
Doubly and Triply Augmented and Diminished Intervals
Intervals can be further contracted or expanded outside of the augmented and diminished qualities. An interval a half step larger than an augmented interval is a doubly augmented interval, while an interval a half step larger than a doubly augmented interval is a triply augmented interval. Likewise, an interval a half step smaller than a diminished interval is a doubly diminished interval, while an interval a half step smaller than a doubly diminished interval is a triply diminished interval.
Compound Intervals
The intervals discussed above, from unison to octave, are simple intervals, which have a size of an octave or smaller. Any interval larger than an octave is a compound interval. In Example 9, the notes A and C first form a minor third (a simple interval). When the C is brought up an octave in the second pair of notes, the interval becomes a minor tenth (a compound interval). Quality remains the same for simple intervals and their corresponding compound intervals.
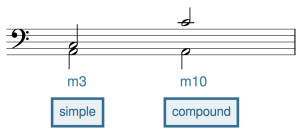
If you want to make a simple interval a compound interval, add 7 to its size. Consequently:
- Unisons (which get the number 1) become octaves (8s)
- 2nds become 9ths
- 3rds become 10ths
- 4ths become 11ths
- 5ths become 12ths
- 6ths become 13ths
These are the most common compound intervals that you will encounter in your music studies. Remember that octaves, 11ths, and 12ths are perfect like their simple counterparts, while 9ths, 10ths, and 13ths are major/minor.
Intervallic Inversion
Intervallic inversion occurs when two notes are “flipped.” In Example 10, for instance, an interval with C on the bottom and E on the top is inverted by moving the C up by an octave. You might be wondering: why is this important? There are two reasons: first, because inverted pairs of notes share many interesting properties (which are sometimes exploited by composers), and second, because inverting a pair of notes can help you to identify or write an interval when you do not want to work from the given bottom note.

Let’s start with the first point: the interesting properties. First, the size of inverted pairs always adds up to 9:
- Unisons (1s) invert to octaves (8s) (1 + 8 = 9) and octaves invert to unisons.
- Seconds invert to sevenths (2 + 7 = 9) and sevenths invert to seconds.
- Thirds invert to sixths (3 + 6 = 9) and sixths invert to thirds.
- Fourths invert to fifths (4 + 5 = 9) and fifths invert to fourths.
Qualities of inverted pairs of notes are also very consistent:
- Perfect intervals invert to perfect intervals.
- Major intervals invert to minor intervals (and minor intervals to major intervals).
- Augmented intervals invert to diminished intervals (and diminished intervals to augmented intervals).
With that information, you can now calculate the inversions of intervals without even looking at staff paper. For example: a major seventh inverts to a minor second, an augmented sixth inverts to a diminished third, and a perfect fourth inverts to a perfect fifth.
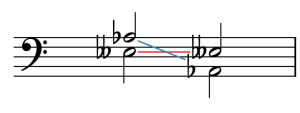
Now for the second point: sometimes you will come across an interval that you do not want to calculate or identify from the bottom note. In the interval E𝄫–A♭ written in Example 11, for instance, identifying the interval using the “Major Scale” method would not work—the bottom note is E𝄫, and there is no key signature for this note (its key signature is “imaginary”). So, if you were given this interval to identify, you might consider inverting the interval. Now the inversion of the interval can be calculated from the non-imaginary key of A♭ major. The key of A♭ major has four flats (B♭, E♭, A♭, and D♭). An E♭ above A♭ would therefore be a perfect fifth; however, this interval has been contracted (made a half step smaller) because the E♭ has been lowered to E𝄫. That means this interval is a d5 (diminished fifth).
Now that we know the inversion of the first interval is a d5, we can calculate the original interval. A diminished fifth inverts to an augmented fourth (because diminished intervals invert to augmented intervals and because five plus four equals nine). Thus, the first interval is an augmented fourth (A4).
Consonance and Dissonance
Intervals are categorized as consonant or dissonant. Consonant intervals are intervals that are considered more stable, as if they do not need to resolve, while dissonant intervals are considered less stable, as if they do need to resolve. These categorizations have varied with milieu. Example 12 shows a table of melodically consonant and dissonant intervals:
[table “63” not found /]
Example 12. Melodically consonant and dissonant intervals.
Example 13 shows harmonically consonant and dissonant intervals:
[table “64” not found /]
Example 13. Harmonically consonant and dissonant intervals.
The implications of consonant and dissonant intervals are discussed further in the Introduction to Species Counterpoint.
Another Method for Intervals: The White-Key Method
Ultimately, intervals need to be committed to memory, both aurally and visually. There are, however, a few tricks to learning how to do this quickly. One such trick is the so-called “white-key method,” which refers to the piano keyboard.
This method requires you to memorize all of the intervals found between the white keys on the piano (or simply all of the intervals in the key of C major). Once you’ve learned these, any interval can be calculated as an alteration of a white-key interval. For example, we can figure out the interval for the notes D and F♯ if we know that the interval D to F is a minor third and this interval has been made one semitone larger: a major third.
Conveniently, there is a lot of repetition of interval size and quality among white-key intervals, summarized in Example 14. Memorize the most frequent type and the exceptions.
- All of the seconds are major except for two: E–F and B–C.
- All of the thirds are minor except for three: C–E, F–A, and G–B, which are major.
- All of the fourths are perfect except for one: F–B, which is an augmented fourth (a tritone).
https://musescore.com/user/32728834/scores/6836757/embed
Example 14. White-key seconds, thirds, and fourths.
Believe it or not, you now know all of the white-key intervals, as long as you understand the concept of intervallic inversion, which was previously explained. For example, if you know that all seconds are major except for E–F and B–C (which are minor), then you know that all sevenths are minor except for F–E and C–B (which are major), as seen in Example 15.

Once you’ve mastered the white-key intervals, you can figure out any other interval by taking into account any accidentals applied to the notes.
Intervallic Enharmonic Equivalence
Example 16 may be useful when thinking about enharmonic equivalence of intervals. In this chart, the columns are different intervallic sizes, while the rows present intervals based on the number of half steps they contain. Each row in this chart is enharmonically equivalent. For example, a major second (ma2) and diminished third (d3) are enharmonically equivalent (both are two half steps). Likewise, an augmented fourth (A4) and diminished fifth (d5) are enharmonically equivalent—both are six half steps in size.
| number of semitones | unis. | 2nd | 3rd | 4th | 5th | 6th | 7th | oct. |
|---|---|---|---|---|---|---|---|---|
| 0 | P1 | d2 | ||||||
| 1 | A1 | mi2 | ||||||
| 2 | ma2 | d3 | ||||||
| 3 | A2 | mi3 | ||||||
| 4 | ma3 | d4 | ||||||
| 5 | A3 | P4 | ||||||
| 6 | A4 | d5 | ||||||
| 7 | P5 | d6 | ||||||
| 8 | A5 | mi6 | ||||||
| 9 | ma6 | d7 | ||||||
| 10 | A6 | mi7 | ||||||
| 11 | ma7 | d8 | ||||||
| 12 | A7 | P8 |
Example 16. Enharmonic equivalence of intervals.
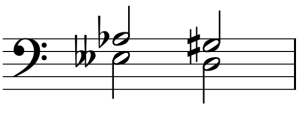
Intervallic enharmonic equivalence is useful when you come across an interval that you do not want to calculate or identify from the bottom note. We have already discussed one method for this situation previously, which was intervallic inversion. You may prefer one method or the other, though both will yield the same result. Example 17 reproduces the interval from Example 11. As you’ll recall, there is no key signature for the bottom note (E𝄫), making identification of this interval difficult. By using enharmonic equivalence, however, we can identify this interval more easily, recognizing that E𝄫 is enharmonically equivalent with D and that A♭ is enharmonically equivalent with G♯. Now we can identify the interval as an A4 (augmented fourth), using the key signature of the enharmonically equivalent bottom note (D).
- Specific Intervals (musictheory.net)
- Interval Introduction (Robert Hutchinson)
- Intervals (Hello Music Theory)
- Diminished and Augmented Intervals (Open Textbooks)
- Diminished and Augmented Intervals (Robert Hutchinson)
- Compound Intervals (Hello Music Theory)
- Interval Inversion (musictheory.net)
- Interval Ear Training (musictheory.net)
- Interval Ear Training (Tone Dear)
- Interval Ear Training (teoria)
- Interval Identification (musictheory.net)
- Keyboard Interval Identification (musictheory.net)
- Interval Identification (.pdf, .pdf, .pdf), in Major Keys (.pdf), in Minor Keys (.pdf)
- Interval Identification and Construction, pp. 18–19 (.pdf)
- Interval Construction (.pdf, .pdf)
- Compound Intervals, pp. 15–17 (.pdf)
- Writing and Identifying Intervals Assignment #1 (.pdf, .mcsz)
- Writing and Identifying Intervals Assignment #2 (.pdf, .mcsz)
- Writing and Identifying Intervals Assignment #3 (.pdf, .mcsz)
The distance between two notes.
.front-matter h6 {
font-size: 1.25em;
}
.textbox--sidebar {
float: right;
margin: 1em 0 1em 1em;
max-width: 45%;
}
.textbox.textbox--key-takeaways .textbox__header p {
font: sans-serif !important;
font-weight: bold;
text-transform: uppercase;
font-style: normal;
font-size: larger;
}
a {
text-decoration: none;
color: blue;
text-transform: uppercase;
}
.front-matter h6 {
font-size: 1.25em;
}
.textbox--sidebar {
float: right;
margin: 1em 0 1em 1em;
max-width: 45%;
}
.textbox.textbox--key-takeaways .textbox__header p {
font: sans-serif !important;
font-weight: bold;
text-transform: uppercase;
font-style: normal;
font-size: larger;
}
a {
color: blue;
text-transform: uppercase;
text-decoration: none;
}
.front-matter h6 {
font-size: 1.25em;
}
.textbox--sidebar {
float: right;
margin: 1em 0 1em 1em;
max-width: 45%;
}
.textbox.textbox--key-takeaways .textbox__header p {
font: sans-serif !important;
font-weight: bold;
text-transform: uppercase;
font-style: normal;
font-size: larger;
}
a {
color: blue;
text-decoration: none;
background-color: green;
}
The number of scale steps between notes of a collection or scale.
.front-matter h6 {
font-size: 1.25em;
}
.textbox--sidebar {
float: right;
margin: 1em 0 1em 1em;
max-width: 45%;
}
.textbox.textbox--key-takeaways .textbox__header p {
font: sans-serif !important;
font-weight: bold;
text-transform: uppercase;
font-style: normal;
font-size: larger;
}
a:link {
text-decoration: none;
}
button {
background: green;
}
.front-matter h6 {
font-size: 1.25em;
}
.textbox--sidebar {
float: right;
margin: 1em 0 1em 1em;
max-width: 45%;
}
.textbox.textbox--key-takeaways .textbox__header p {
font: sans-serif !important;
font-weight: bold;
text-transform: uppercase;
font-style: normal;
font-size: larger;
}
a:link {
text-decoration: none;
}
.front-matter h6 {
font-size: 1.25em;
}
.textbox--sidebar {
float: right;
margin: 1em 0 1em 1em;
max-width: 45%;
}
.textbox.textbox--key-takeaways .textbox__header p {
font: sans-serif !important;
font-weight: bold;
text-transform: uppercase;
font-style: normal;
font-size: larger;
}
a:link {
text-decoration: none;
}
button:a {
background: green;
}
An interval that is larger than an octave.
Key Takeaways
- A beat is a pulse in music that regularly recurs.
- Simple meters are meters in which the beat divides into two, and then further subdivides into four.
- Duple meters have groupings of two beats, triple meters have groupings of three beats, and quadruple meters have groupings of four beats.
- There are different conducting patterns for duple, triple, and quadruple meters.
- A measure is equivalent to one group of beats (duple, triple, or quadruple). Measures are separated by bar lines.
- Time signatures in simple meters express two things: how many beats are contained in each measure (the top number), and the beat unit (the bottom number), which refers to the note value that is the beat.
- A beam visually connects notes together, grouping them by beat. Beaming changes in different time signatures.
- Notes below the middle line on a staff are up-stemmed, while notes above the middle line on a staff are down-stemmed. Flag direction works similarly.
In Rhythmic and Rest Values, we discussed the different rhythmic values of notes and rests. Musicians organize rhythmic values into various meters, which are—broadly speaking—formed as the result of recurrent patterns of accents in musical performances.
Terminology
Listen to the following performance by the contemporary musical group Postmodern Jukebox ( Example 1). They are performing a cover of the song "Wannabe" by the Spice Girls (originally released in 1996). Beginning at 0:11, it is easy to tap or clap along to this recording. What you are tapping along to is called a beat—a pulse in music that regularly recurs.
Example 1. A cover of "Wannabe" performed by Postmodern Jukebox; listen starting at 0:11.
Example 1 is in a simple meter: a meter in which the beat divides into two, and then further subdivides into four. You can feel this yourself by tapping your beat twice as fast; you might also think of this as dividing your beat into two smaller beats.
Different numbers of beats group into different meters. Duple meters contain beats that are grouped into twos, while Triple meters contain beats that are grouped into threes, and Quadruple meters contain beats that are grouped into fours.
Listening to Simple Meters
Let's listen to examples of simple duple, simple triple, and simple quadruple meters. A simple duple meter contains two beats, each of which divides into two (and further subdivides into four). "The Stars and Stripes Forever" (1896), written by John Philip Sousa, is in a simple duple meter.
Listen to Example 2, and tap along, feeling how the beats group into sets of two:
Example 2. "The Stars and Stripes Forever" played by the Dallas Winds.
A simple triple meter contains three beats, each of which divides into two (and further subdivides into four). Wolfgang Amadeus Mozart's "Minuet in F major," K.2 (1774) is in a simple triple meter. Listen to Example 3, and tap along, feeling how the beats group into sets of three:
Example 3. Mozart's "Minuet in F major," played by Alan Huckleberry.
Finally, a simple quadruple meter contains four beats, each of which divides into two (and further subdivides into four). The song "Cake" (2017) by Flo Rida is in a simple quadruple meter. Listen to Example 4 starting at 0:45 and tap along, feeling how the beats group into sets of four:
Example 4. "Cake" by Flo Rida; listen starting at 0:45.
As you can hear and feel (by tapping along), musical compositions in a wide variety of styles are governed by meter. You might practice identifying the meters of some of your favorite songs or musical compositions as simple duple, simple triple, or simple quadruple; listening carefully and tapping along is the best way to do this. Note that simple quadruple meters feel similar to simple duple meters, since four beats can be divided into two groups of two beats. It may not always be immediately apparent if a work is in a simple duple or simple quadruple meter by listening alone.
Conducting Patterns
If you have ever sung in a choir or played an instrument in a band or orchestra, then you have likely had experience with a conductor. Conductors have many jobs. One of these jobs is to provide conducting patterns for the musicians in their choir, band, or orchestra. Conducting patterns serve two main purposes: first, they establish a tempo, and second, they establish a meter.
The three most common conducting patterns outline duple, triple, and quadruple meters. Duple meters are conducted with a downward/outward motion (step 1), followed by an upward motion (step 2), as seen in Example 5. Triple meters are conducted with a downward motion (step 1), an outward motion (step 2), and an upward motion (step 3), as seen in Example 6. Quadruple meters are conducted with a downward motion (step 1), an inward motion (step 2), an outward motion (step 3), and an upward motion (step 4), as seen in Example 7:
Beat 1 of each of these measures is considered a downbeat . A downbeat is conducted with a downward motion, and you may hear and feel that it has more "weight" or "heaviness" then the other beats. An upbeat is the last beat of any measure. Upbeats are conducted with an upward motion, and you may feel and hear that they are anticipatory in nature.
Example 8 shows a short video demonstrating these three conducting patterns:
Example 8. Dr. John Lopez (Texas A&M University, Kingsville) demonstrates duple, triple, and quadruple conducting patterns.
You can practice these conducting patterns while listening to Example 2 (duple), Example 3 (triple), and Example 4 (quadruple) above.
Time Signatures
In Western musical notation, beat groupings (duple, triple, quadruple, etc.) are shown using bar lines, which separate music into measures (also called bars), as shown in Example 9. Each measure is equivalent to one beat grouping.

In simple meters, time signatures (also called meter signatures) express two things: 1) how many beats are contained in each measure, and 2) the beat unit (which note value gets the beat). Time signatures are expressed by two numbers, one above the other, placed after the clef (Example 10).

A time signature is not a fraction, though it may look like one; note that there is no line between the two numbers. In simple meters, the top number of a time signature represents the number of beats in each measure, while the bottom number represents the beat unit.
In simple meters, the top number is always 2, 3, or 4, corresponding to duple, triple, or quadruple beat patterns. The bottom number is usually one of the following:
- 2, which means the half note gets the beat.
- 4, which means the quarter note gets the beat.
- 8, which means the eighth note gets the beat.
You may also see the bottom number 16 (the sixteenth note gets the beat) or 1 (the whole note gets the beat) in simple meter time signatures.
There are two additional simple meter time signatures, which are 𝄴 (common time) and 𝄵 (cut time). Common time is the equivalent of [latex]\mathbf{^4_4}[/latex] (simple quadruple—four beats per measure), while cut time is the equivalent of [latex]\mathbf{^2_2}[/latex] (simple duple—two beats per measure).
Counting in Simple Meter
Counting rhythms aloud is important for musical performance; as a singer or instrumentalist, you must be able to perform rhythms that are written in Western musical notation. Conducting while counting rhythms is highly recommended and will help you to keep a steady tempo. Please note that your instructor may employ a different counting system. Open Music Theory privileges American traditional counting, but this is not the only method.
Example 11 shows a rhythm in a [latex]\mathbf{^4_4}[/latex] time signature, which is a simple quadruple meter. This time signature means that there are four beats per measure (the top 4) and that the quarter note gets the beat (the bottom 4).
- In each measure, each quarter note gets a count, expressed with Arabic numerals—"1, 2, 3, 4."
- When notes last longer than one beat (such as a half or whole note in this example), the count is held over multiple beats. Beats that are not counted out loud are written in parentheses.
- When the beat in a simple meter is divided into two, the divisions are counted aloud with the syllable “and,” which is usually notated with the plus sign (+). So, if the quarter note gets the beat, the second eighth note in each beat would be counted as “and.”
- Further subdivisions at the sixteenth-note level are counted as “e” (pronounced as a long vowel, as in the word “see”) and “a” (pronounced “uh”). At the thirty-second-note level, further subdivisions add the syllable “ta” in between each of the previous syllables.
Example 11. Rhythm in 4/4 time.
Simple duple meters have only two beats and simple triple meters have only three, but the subdivisions are counted the same way (Example 12).
Example 12. Simple duple meters have two beats per measure; simple triple meters have three.
Like with notes that last for two or more beats, beats that are not articulated because of rests, ties, and dots are also not counted out loud. These beats are usually written in parentheses, as shown in Example 13:
Example 13. Beats that are not counted out loud are put in parentheses.

When an example begins with a pickup note (anacrusis), your count will not begin on "1," as shown in Example 14. An anacrusis is counted as the last note(s) of an imaginary measure. When a work begins with an anacrusis, the last measure is usually shortened by the length of the anacrusis. This is demonstrated in Example 14: the anacrusis is one quarter note in length, so the last measure is only three beats long (i.e., it is missing one quarter note).
Counting with Beat Units of 2, 8, and 16
In simple meters with other beat units (shown in the bottom number of the time signature), the same counting patterns are used for the beats and subdivisions, but they correspond to different note values. Example 15 shows a rhythm with a [latex]\mathbf{^4_4}[/latex] time signature, followed by the same rhythms with different beat units. Each of these rhythms sounds the same and is counted the same. They are also all considered simple quadruple meters. The difference in each example is the bottom number of the time signature—which note gets the beat unit (quarter, half, eighth, or sixteenth).
Example 15. The same counted rhythm, as written in a meter with (a) a quarter-note beat, (b) a half-note beat, (c) an eighth-note beat, and (d) a sixteenth-note beat.
Beaming, Stems, Flags, and Multi-Measure Rests
Beams connect notes together by beat. As Example 16 shows, this means that beaming changes depending on the time signature. In the first measure, sixteenth notes are grouped into sets of four, because four sixteenth notes in a [latex]\mathbf{^4_4}[/latex] time signature are equivalent to one beat. In the second measure, however, sixteenth notes are grouped into sets of two, because one beat in a [latex]\mathbf{^4_8}[/latex] time signature is only equivalent to two sixteenth notes.
Example 16. Beaming in two different meters.

Note that in vocal music, beaming is sometimes only used to connect notes sung on the same syllable. If you are accustomed to music without beaming, you may need to pay special attention to beaming conventions until you have mastered them. In the top staff of Example 17, the eighth notes are not grouped with beams, making it difficult to see where beats 2 and 3 in the triple meter begin. The bottom staff shows that if we re-notate the rhythm so that the notes that fall within the same beat are grouped together with a beam, it makes the music much easier to read. Note that these two rhythms sound the same, even though they are beamed differently. The ability to group events according to a hierarchy is an important part of human perception, which is why beaming helps us visually parse notated musical rhythms—the metrical structure provides a hierarchy that we show using notational tools like beaming.
Example 18 shows several different note values beamed together to show the beat unit. The first line does not require beams because quarter notes are never beamed, but all subsequent lines do need beams to clarify beats.


The second measure of Example 19 shows that when notes are grouped together with beams, the stem direction is determined by the note farthest from the middle line. On beat 1 of measure 2, this note is E5, which is above the middle line, so down-stems are used. Beat 2 uses up-stems because the note farthest from the middle line is the E4 below it.

Flagging is determined by stem direction (Example 20). Notes above the middle line receive a down-stem (on the left) and an inward-facing flag (facing right). Notes below the middle line receive an up-stem (on the right) and an outward-facing flag (facing left). Notes on the middle line can be flagged in either direction, usually depending on the contour of the musical line.
Partial beams can be used for mixed rhythmic groupings, as shown in Example 21. Sometimes these beaming conventions look strange to students who have had less experience with reading beamed music. If this is the case, you will want to pay special attention to how the notes in Example 21 are beamed.
Example 21. Partial beams are used for some mixed rhythmic groupings.

Rests that last for multiple full measures are sometimes notated as seen in Example 22. This example indicates that the musician is to rest for a duration of four full measures.
A Note on Ties
We have already encountered ties that can be used to extend a note over a measure line. But ties can also be used like beams to clarify the metrical structure within a measure. In the first measure of Example 23, beat 2 begins in the middle of the eighth note, making it difficult to see the metrical structure. Breaking the eighth note into two sixteenth notes connected by a tie, as shown in the second measure, clearly shows the beginning of beat 2.

- Simple Meter Tutorial (musictheory.net)
- Video Tutorial on Simple Meter, Beats, and Beaming (YouTube)
- Conducting Patterns (YouTube)
- Simple Meter Time Signatures (liveabout.com)
- Video Tutorial on Counting Simple Meters (One Minute Music Lessons)
- Simple Meter Counting (YouTube)
- Beaming Rules (Music Notes Now)
- Beaming Examples (Dr. Sebastian Anthony Birch)
- Time Signatures and Rhythms (.pdf)
- Terminology, Bar Lines, Fill-in-rhythms, Re-beaming (.pdf)
- Meters, Time Signatures, Re-beaming (website)
- Bar Lines, Time Signatures, Counting (.pdf)
- Time Signatures, Re-beaming, p. 4 (.pdf)
- Fill-in-rhythms (.pdf)
- Time Signatures (.pdf, .pdf)
- Bar Lines (.pdf, .pdf, .pdf)
Key Takeaways
- A beat is a pulse in music that regularly recurs.
- Simple meters are meters in which the beat divides into two, and then further subdivides into four.
- Duple meters have groupings of two beats, triple meters have groupings of three beats, and quadruple meters have groupings of four beats.
- There are different conducting patterns for duple, triple, and quadruple meters.
- A measure is equivalent to one group of beats (duple, triple, or quadruple). Measures are separated by bar lines.
- Time signatures in simple meters express two things: how many beats are contained in each measure (the top number), and the beat unit (the bottom number), which refers to the note value that is the beat.
- A beam visually connects notes together, grouping them by beat. Beaming changes in different time signatures.
- Notes below the middle line on a staff are up-stemmed, while notes above the middle line on a staff are down-stemmed. Flag direction works similarly.
In Rhythmic and Rest Values, we discussed the different rhythmic values of notes and rests. Musicians organize rhythmic values into various meters, which are—broadly speaking—formed as the result of recurrent patterns of accents in musical performances.
Terminology
Listen to the following performance by the contemporary musical group Postmodern Jukebox ( Example 1). They are performing a cover of the song "Wannabe" by the Spice Girls (originally released in 1996). Beginning at 0:11, it is easy to tap or clap along to this recording. What you are tapping along to is called a beat—a pulse in music that regularly recurs.
Example 1. A cover of "Wannabe" performed by Postmodern Jukebox; listen starting at 0:11.
Example 1 is in a simple meter: a meter in which the beat divides into two, and then further subdivides into four. You can feel this yourself by tapping your beat twice as fast; you might also think of this as dividing your beat into two smaller beats.
Different numbers of beats group into different meters. Duple meters contain beats that are grouped into twos, while Triple meters contain beats that are grouped into threes, and Quadruple meters contain beats that are grouped into fours.
Listening to Simple Meters
Let's listen to examples of simple duple, simple triple, and simple quadruple meters. A simple duple meter contains two beats, each of which divides into two (and further subdivides into four). "The Stars and Stripes Forever" (1896), written by John Philip Sousa, is in a simple duple meter.
Listen to Example 2, and tap along, feeling how the beats group into sets of two:
Example 2. "The Stars and Stripes Forever" played by the Dallas Winds.
A simple triple meter contains three beats, each of which divides into two (and further subdivides into four). Wolfgang Amadeus Mozart's "Minuet in F major," K.2 (1774) is in a simple triple meter. Listen to Example 3, and tap along, feeling how the beats group into sets of three:
Example 3. Mozart's "Minuet in F major," played by Alan Huckleberry.
Finally, a simple quadruple meter contains four beats, each of which divides into two (and further subdivides into four). The song "Cake" (2017) by Flo Rida is in a simple quadruple meter. Listen to Example 4 starting at 0:45 and tap along, feeling how the beats group into sets of four:
Example 4. "Cake" by Flo Rida; listen starting at 0:45.
As you can hear and feel (by tapping along), musical compositions in a wide variety of styles are governed by meter. You might practice identifying the meters of some of your favorite songs or musical compositions as simple duple, simple triple, or simple quadruple; listening carefully and tapping along is the best way to do this. Note that simple quadruple meters feel similar to simple duple meters, since four beats can be divided into two groups of two beats. It may not always be immediately apparent if a work is in a simple duple or simple quadruple meter by listening alone.
Conducting Patterns
If you have ever sung in a choir or played an instrument in a band or orchestra, then you have likely had experience with a conductor. Conductors have many jobs. One of these jobs is to provide conducting patterns for the musicians in their choir, band, or orchestra. Conducting patterns serve two main purposes: first, they establish a tempo, and second, they establish a meter.
The three most common conducting patterns outline duple, triple, and quadruple meters. Duple meters are conducted with a downward/outward motion (step 1), followed by an upward motion (step 2), as seen in Example 5. Triple meters are conducted with a downward motion (step 1), an outward motion (step 2), and an upward motion (step 3), as seen in Example 6. Quadruple meters are conducted with a downward motion (step 1), an inward motion (step 2), an outward motion (step 3), and an upward motion (step 4), as seen in Example 7:
Beat 1 of each of these measures is considered a downbeat . A downbeat is conducted with a downward motion, and you may hear and feel that it has more "weight" or "heaviness" then the other beats. An upbeat is the last beat of any measure. Upbeats are conducted with an upward motion, and you may feel and hear that they are anticipatory in nature.
Example 8 shows a short video demonstrating these three conducting patterns:
Example 8. Dr. John Lopez (Texas A&M University, Kingsville) demonstrates duple, triple, and quadruple conducting patterns.
You can practice these conducting patterns while listening to Example 2 (duple), Example 3 (triple), and Example 4 (quadruple) above.
Time Signatures
In Western musical notation, beat groupings (duple, triple, quadruple, etc.) are shown using bar lines, which separate music into measures (also called bars), as shown in Example 9. Each measure is equivalent to one beat grouping.

In simple meters, time signatures (also called meter signatures) express two things: 1) how many beats are contained in each measure, and 2) the beat unit (which note value gets the beat). Time signatures are expressed by two numbers, one above the other, placed after the clef (Example 10).

A time signature is not a fraction, though it may look like one; note that there is no line between the two numbers. In simple meters, the top number of a time signature represents the number of beats in each measure, while the bottom number represents the beat unit.
In simple meters, the top number is always 2, 3, or 4, corresponding to duple, triple, or quadruple beat patterns. The bottom number is usually one of the following:
- 2, which means the half note gets the beat.
- 4, which means the quarter note gets the beat.
- 8, which means the eighth note gets the beat.
You may also see the bottom number 16 (the sixteenth note gets the beat) or 1 (the whole note gets the beat) in simple meter time signatures.
There are two additional simple meter time signatures, which are 𝄴 (common time) and 𝄵 (cut time). Common time is the equivalent of [latex]\mathbf{^4_4}[/latex] (simple quadruple—four beats per measure), while cut time is the equivalent of [latex]\mathbf{^2_2}[/latex] (simple duple—two beats per measure).
Counting in Simple Meter
Counting rhythms aloud is important for musical performance; as a singer or instrumentalist, you must be able to perform rhythms that are written in Western musical notation. Conducting while counting rhythms is highly recommended and will help you to keep a steady tempo. Please note that your instructor may employ a different counting system. Open Music Theory privileges American traditional counting, but this is not the only method.
Example 11 shows a rhythm in a [latex]\mathbf{^4_4}[/latex] time signature, which is a simple quadruple meter. This time signature means that there are four beats per measure (the top 4) and that the quarter note gets the beat (the bottom 4).
- In each measure, each quarter note gets a count, expressed with Arabic numerals—"1, 2, 3, 4."
- When notes last longer than one beat (such as a half or whole note in this example), the count is held over multiple beats. Beats that are not counted out loud are written in parentheses.
- When the beat in a simple meter is divided into two, the divisions are counted aloud with the syllable “and,” which is usually notated with the plus sign (+). So, if the quarter note gets the beat, the second eighth note in each beat would be counted as “and.”
- Further subdivisions at the sixteenth-note level are counted as “e” (pronounced as a long vowel, as in the word “see”) and “a” (pronounced “uh”). At the thirty-second-note level, further subdivisions add the syllable “ta” in between each of the previous syllables.
Example 11. Rhythm in 4/4 time.
Simple duple meters have only two beats and simple triple meters have only three, but the subdivisions are counted the same way (Example 12).
Example 12. Simple duple meters have two beats per measure; simple triple meters have three.
Like with notes that last for two or more beats, beats that are not articulated because of rests, ties, and dots are also not counted out loud. These beats are usually written in parentheses, as shown in Example 13:
Example 13. Beats that are not counted out loud are put in parentheses.

When an example begins with a pickup note (anacrusis), your count will not begin on "1," as shown in Example 14. An anacrusis is counted as the last note(s) of an imaginary measure. When a work begins with an anacrusis, the last measure is usually shortened by the length of the anacrusis. This is demonstrated in Example 14: the anacrusis is one quarter note in length, so the last measure is only three beats long (i.e., it is missing one quarter note).
Counting with Beat Units of 2, 8, and 16
In simple meters with other beat units (shown in the bottom number of the time signature), the same counting patterns are used for the beats and subdivisions, but they correspond to different note values. Example 15 shows a rhythm with a [latex]\mathbf{^4_4}[/latex] time signature, followed by the same rhythms with different beat units. Each of these rhythms sounds the same and is counted the same. They are also all considered simple quadruple meters. The difference in each example is the bottom number of the time signature—which note gets the beat unit (quarter, half, eighth, or sixteenth).
Example 15. The same counted rhythm, as written in a meter with (a) a quarter-note beat, (b) a half-note beat, (c) an eighth-note beat, and (d) a sixteenth-note beat.
Beaming, Stems, Flags, and Multi-Measure Rests
Beams connect notes together by beat. As Example 16 shows, this means that beaming changes depending on the time signature. In the first measure, sixteenth notes are grouped into sets of four, because four sixteenth notes in a [latex]\mathbf{^4_4}[/latex] time signature are equivalent to one beat. In the second measure, however, sixteenth notes are grouped into sets of two, because one beat in a [latex]\mathbf{^4_8}[/latex] time signature is only equivalent to two sixteenth notes.
Example 16. Beaming in two different meters.

Note that in vocal music, beaming is sometimes only used to connect notes sung on the same syllable. If you are accustomed to music without beaming, you may need to pay special attention to beaming conventions until you have mastered them. In the top staff of Example 17, the eighth notes are not grouped with beams, making it difficult to see where beats 2 and 3 in the triple meter begin. The bottom staff shows that if we re-notate the rhythm so that the notes that fall within the same beat are grouped together with a beam, it makes the music much easier to read. Note that these two rhythms sound the same, even though they are beamed differently. The ability to group events according to a hierarchy is an important part of human perception, which is why beaming helps us visually parse notated musical rhythms—the metrical structure provides a hierarchy that we show using notational tools like beaming.
Example 18 shows several different note values beamed together to show the beat unit. The first line does not require beams because quarter notes are never beamed, but all subsequent lines do need beams to clarify beats.


The second measure of Example 19 shows that when notes are grouped together with beams, the stem direction is determined by the note farthest from the middle line. On beat 1 of measure 2, this note is E5, which is above the middle line, so down-stems are used. Beat 2 uses up-stems because the note farthest from the middle line is the E4 below it.

Flagging is determined by stem direction (Example 20). Notes above the middle line receive a down-stem (on the left) and an inward-facing flag (facing right). Notes below the middle line receive an up-stem (on the right) and an outward-facing flag (facing left). Notes on the middle line can be flagged in either direction, usually depending on the contour of the musical line.
Partial beams can be used for mixed rhythmic groupings, as shown in Example 21. Sometimes these beaming conventions look strange to students who have had less experience with reading beamed music. If this is the case, you will want to pay special attention to how the notes in Example 21 are beamed.
Example 21. Partial beams are used for some mixed rhythmic groupings.

Rests that last for multiple full measures are sometimes notated as seen in Example 22. This example indicates that the musician is to rest for a duration of four full measures.
A Note on Ties
We have already encountered ties that can be used to extend a note over a measure line. But ties can also be used like beams to clarify the metrical structure within a measure. In the first measure of Example 23, beat 2 begins in the middle of the eighth note, making it difficult to see the metrical structure. Breaking the eighth note into two sixteenth notes connected by a tie, as shown in the second measure, clearly shows the beginning of beat 2.

- Simple Meter Tutorial (musictheory.net)
- Video Tutorial on Simple Meter, Beats, and Beaming (YouTube)
- Conducting Patterns (YouTube)
- Simple Meter Time Signatures (liveabout.com)
- Video Tutorial on Counting Simple Meters (One Minute Music Lessons)
- Simple Meter Counting (YouTube)
- Beaming Rules (Music Notes Now)
- Beaming Examples (Dr. Sebastian Anthony Birch)
- Time Signatures and Rhythms (.pdf)
- Terminology, Bar Lines, Fill-in-rhythms, Re-beaming (.pdf)
- Meters, Time Signatures, Re-beaming (website)
- Bar Lines, Time Signatures, Counting (.pdf)
- Time Signatures, Re-beaming, p. 4 (.pdf)
- Fill-in-rhythms (.pdf)
- Time Signatures (.pdf, .pdf)
- Bar Lines (.pdf, .pdf, .pdf)
Key Takeaways
- A beat is a pulse in music that regularly recurs.
- Simple meters are meters in which the beat divides into two, and then further subdivides into four.
- Duple meters have groupings of two beats, triple meters have groupings of three beats, and quadruple meters have groupings of four beats.
- There are different conducting patterns for duple, triple, and quadruple meters.
- A measure is equivalent to one group of beats (duple, triple, or quadruple). Measures are separated by bar lines.
- Time signatures in simple meters express two things: how many beats are contained in each measure (the top number), and the beat unit (the bottom number), which refers to the note value that is the beat.
- A beam visually connects notes together, grouping them by beat. Beaming changes in different time signatures.
- Notes below the middle line on a staff are up-stemmed, while notes above the middle line on a staff are down-stemmed. Flag direction works similarly.
In Rhythmic and Rest Values, we discussed the different rhythmic values of notes and rests. Musicians organize rhythmic values into various meters, which are—broadly speaking—formed as the result of recurrent patterns of accents in musical performances.
Terminology
Listen to the following performance by the contemporary musical group Postmodern Jukebox ( Example 1). They are performing a cover of the song "Wannabe" by the Spice Girls (originally released in 1996). Beginning at 0:11, it is easy to tap or clap along to this recording. What you are tapping along to is called a beat—a pulse in music that regularly recurs.
Example 1. A cover of "Wannabe" performed by Postmodern Jukebox; listen starting at 0:11.
Example 1 is in a simple meter: a meter in which the beat divides into two, and then further subdivides into four. You can feel this yourself by tapping your beat twice as fast; you might also think of this as dividing your beat into two smaller beats.
Different numbers of beats group into different meters. Duple meters contain beats that are grouped into twos, while Triple meters contain beats that are grouped into threes, and Quadruple meters contain beats that are grouped into fours.
Listening to Simple Meters
Let's listen to examples of simple duple, simple triple, and simple quadruple meters. A simple duple meter contains two beats, each of which divides into two (and further subdivides into four). "The Stars and Stripes Forever" (1896), written by John Philip Sousa, is in a simple duple meter.
Listen to Example 2, and tap along, feeling how the beats group into sets of two:
Example 2. "The Stars and Stripes Forever" played by the Dallas Winds.
A simple triple meter contains three beats, each of which divides into two (and further subdivides into four). Wolfgang Amadeus Mozart's "Minuet in F major," K.2 (1774) is in a simple triple meter. Listen to Example 3, and tap along, feeling how the beats group into sets of three:
Example 3. Mozart's "Minuet in F major," played by Alan Huckleberry.
Finally, a simple quadruple meter contains four beats, each of which divides into two (and further subdivides into four). The song "Cake" (2017) by Flo Rida is in a simple quadruple meter. Listen to Example 4 starting at 0:45 and tap along, feeling how the beats group into sets of four:
Example 4. "Cake" by Flo Rida; listen starting at 0:45.
As you can hear and feel (by tapping along), musical compositions in a wide variety of styles are governed by meter. You might practice identifying the meters of some of your favorite songs or musical compositions as simple duple, simple triple, or simple quadruple; listening carefully and tapping along is the best way to do this. Note that simple quadruple meters feel similar to simple duple meters, since four beats can be divided into two groups of two beats. It may not always be immediately apparent if a work is in a simple duple or simple quadruple meter by listening alone.
Conducting Patterns
If you have ever sung in a choir or played an instrument in a band or orchestra, then you have likely had experience with a conductor. Conductors have many jobs. One of these jobs is to provide conducting patterns for the musicians in their choir, band, or orchestra. Conducting patterns serve two main purposes: first, they establish a tempo, and second, they establish a meter.
The three most common conducting patterns outline duple, triple, and quadruple meters. Duple meters are conducted with a downward/outward motion (step 1), followed by an upward motion (step 2), as seen in Example 5. Triple meters are conducted with a downward motion (step 1), an outward motion (step 2), and an upward motion (step 3), as seen in Example 6. Quadruple meters are conducted with a downward motion (step 1), an inward motion (step 2), an outward motion (step 3), and an upward motion (step 4), as seen in Example 7:
Beat 1 of each of these measures is considered a downbeat . A downbeat is conducted with a downward motion, and you may hear and feel that it has more "weight" or "heaviness" then the other beats. An upbeat is the last beat of any measure. Upbeats are conducted with an upward motion, and you may feel and hear that they are anticipatory in nature.
Example 8 shows a short video demonstrating these three conducting patterns:
Example 8. Dr. John Lopez (Texas A&M University, Kingsville) demonstrates duple, triple, and quadruple conducting patterns.
You can practice these conducting patterns while listening to Example 2 (duple), Example 3 (triple), and Example 4 (quadruple) above.
Time Signatures
In Western musical notation, beat groupings (duple, triple, quadruple, etc.) are shown using bar lines, which separate music into measures (also called bars), as shown in Example 9. Each measure is equivalent to one beat grouping.

In simple meters, time signatures (also called meter signatures) express two things: 1) how many beats are contained in each measure, and 2) the beat unit (which note value gets the beat). Time signatures are expressed by two numbers, one above the other, placed after the clef (Example 10).

A time signature is not a fraction, though it may look like one; note that there is no line between the two numbers. In simple meters, the top number of a time signature represents the number of beats in each measure, while the bottom number represents the beat unit.
In simple meters, the top number is always 2, 3, or 4, corresponding to duple, triple, or quadruple beat patterns. The bottom number is usually one of the following:
- 2, which means the half note gets the beat.
- 4, which means the quarter note gets the beat.
- 8, which means the eighth note gets the beat.
You may also see the bottom number 16 (the sixteenth note gets the beat) or 1 (the whole note gets the beat) in simple meter time signatures.
There are two additional simple meter time signatures, which are 𝄴 (common time) and 𝄵 (cut time). Common time is the equivalent of [latex]\mathbf{^4_4}[/latex] (simple quadruple—four beats per measure), while cut time is the equivalent of [latex]\mathbf{^2_2}[/latex] (simple duple—two beats per measure).
Counting in Simple Meter
Counting rhythms aloud is important for musical performance; as a singer or instrumentalist, you must be able to perform rhythms that are written in Western musical notation. Conducting while counting rhythms is highly recommended and will help you to keep a steady tempo. Please note that your instructor may employ a different counting system. Open Music Theory privileges American traditional counting, but this is not the only method.
Example 11 shows a rhythm in a [latex]\mathbf{^4_4}[/latex] time signature, which is a simple quadruple meter. This time signature means that there are four beats per measure (the top 4) and that the quarter note gets the beat (the bottom 4).
- In each measure, each quarter note gets a count, expressed with Arabic numerals—"1, 2, 3, 4."
- When notes last longer than one beat (such as a half or whole note in this example), the count is held over multiple beats. Beats that are not counted out loud are written in parentheses.
- When the beat in a simple meter is divided into two, the divisions are counted aloud with the syllable “and,” which is usually notated with the plus sign (+). So, if the quarter note gets the beat, the second eighth note in each beat would be counted as “and.”
- Further subdivisions at the sixteenth-note level are counted as “e” (pronounced as a long vowel, as in the word “see”) and “a” (pronounced “uh”). At the thirty-second-note level, further subdivisions add the syllable “ta” in between each of the previous syllables.
Example 11. Rhythm in 4/4 time.
Simple duple meters have only two beats and simple triple meters have only three, but the subdivisions are counted the same way (Example 12).
Example 12. Simple duple meters have two beats per measure; simple triple meters have three.
Like with notes that last for two or more beats, beats that are not articulated because of rests, ties, and dots are also not counted out loud. These beats are usually written in parentheses, as shown in Example 13:
Example 13. Beats that are not counted out loud are put in parentheses.

When an example begins with a pickup note (anacrusis), your count will not begin on "1," as shown in Example 14. An anacrusis is counted as the last note(s) of an imaginary measure. When a work begins with an anacrusis, the last measure is usually shortened by the length of the anacrusis. This is demonstrated in Example 14: the anacrusis is one quarter note in length, so the last measure is only three beats long (i.e., it is missing one quarter note).
Counting with Beat Units of 2, 8, and 16
In simple meters with other beat units (shown in the bottom number of the time signature), the same counting patterns are used for the beats and subdivisions, but they correspond to different note values. Example 15 shows a rhythm with a [latex]\mathbf{^4_4}[/latex] time signature, followed by the same rhythms with different beat units. Each of these rhythms sounds the same and is counted the same. They are also all considered simple quadruple meters. The difference in each example is the bottom number of the time signature—which note gets the beat unit (quarter, half, eighth, or sixteenth).
Example 15. The same counted rhythm, as written in a meter with (a) a quarter-note beat, (b) a half-note beat, (c) an eighth-note beat, and (d) a sixteenth-note beat.
Beaming, Stems, Flags, and Multi-Measure Rests
Beams connect notes together by beat. As Example 16 shows, this means that beaming changes depending on the time signature. In the first measure, sixteenth notes are grouped into sets of four, because four sixteenth notes in a [latex]\mathbf{^4_4}[/latex] time signature are equivalent to one beat. In the second measure, however, sixteenth notes are grouped into sets of two, because one beat in a [latex]\mathbf{^4_8}[/latex] time signature is only equivalent to two sixteenth notes.
Example 16. Beaming in two different meters.

Note that in vocal music, beaming is sometimes only used to connect notes sung on the same syllable. If you are accustomed to music without beaming, you may need to pay special attention to beaming conventions until you have mastered them. In the top staff of Example 17, the eighth notes are not grouped with beams, making it difficult to see where beats 2 and 3 in the triple meter begin. The bottom staff shows that if we re-notate the rhythm so that the notes that fall within the same beat are grouped together with a beam, it makes the music much easier to read. Note that these two rhythms sound the same, even though they are beamed differently. The ability to group events according to a hierarchy is an important part of human perception, which is why beaming helps us visually parse notated musical rhythms—the metrical structure provides a hierarchy that we show using notational tools like beaming.
Example 18 shows several different note values beamed together to show the beat unit. The first line does not require beams because quarter notes are never beamed, but all subsequent lines do need beams to clarify beats.


The second measure of Example 19 shows that when notes are grouped together with beams, the stem direction is determined by the note farthest from the middle line. On beat 1 of measure 2, this note is E5, which is above the middle line, so down-stems are used. Beat 2 uses up-stems because the note farthest from the middle line is the E4 below it.

Flagging is determined by stem direction (Example 20). Notes above the middle line receive a down-stem (on the left) and an inward-facing flag (facing right). Notes below the middle line receive an up-stem (on the right) and an outward-facing flag (facing left). Notes on the middle line can be flagged in either direction, usually depending on the contour of the musical line.
Partial beams can be used for mixed rhythmic groupings, as shown in Example 21. Sometimes these beaming conventions look strange to students who have had less experience with reading beamed music. If this is the case, you will want to pay special attention to how the notes in Example 21 are beamed.
Example 21. Partial beams are used for some mixed rhythmic groupings.

Rests that last for multiple full measures are sometimes notated as seen in Example 22. This example indicates that the musician is to rest for a duration of four full measures.
A Note on Ties
We have already encountered ties that can be used to extend a note over a measure line. But ties can also be used like beams to clarify the metrical structure within a measure. In the first measure of Example 23, beat 2 begins in the middle of the eighth note, making it difficult to see the metrical structure. Breaking the eighth note into two sixteenth notes connected by a tie, as shown in the second measure, clearly shows the beginning of beat 2.

- Simple Meter Tutorial (musictheory.net)
- Video Tutorial on Simple Meter, Beats, and Beaming (YouTube)
- Conducting Patterns (YouTube)
- Simple Meter Time Signatures (liveabout.com)
- Video Tutorial on Counting Simple Meters (One Minute Music Lessons)
- Simple Meter Counting (YouTube)
- Beaming Rules (Music Notes Now)
- Beaming Examples (Dr. Sebastian Anthony Birch)
- Time Signatures and Rhythms (.pdf)
- Terminology, Bar Lines, Fill-in-rhythms, Re-beaming (.pdf)
- Meters, Time Signatures, Re-beaming (website)
- Bar Lines, Time Signatures, Counting (.pdf)
- Time Signatures, Re-beaming, p. 4 (.pdf)
- Fill-in-rhythms (.pdf)
- Time Signatures (.pdf, .pdf)
- Bar Lines (.pdf, .pdf, .pdf)
A physical and/or social setting.
An ordered collection of half steps (H) and whole steps (W) as follows (ascending): W–W–H–W–W–W–H.
A relationship between notes, intervals, or chords that sound the same but are spelled differently.

